Design of Retaining Wall Using Staad Pro

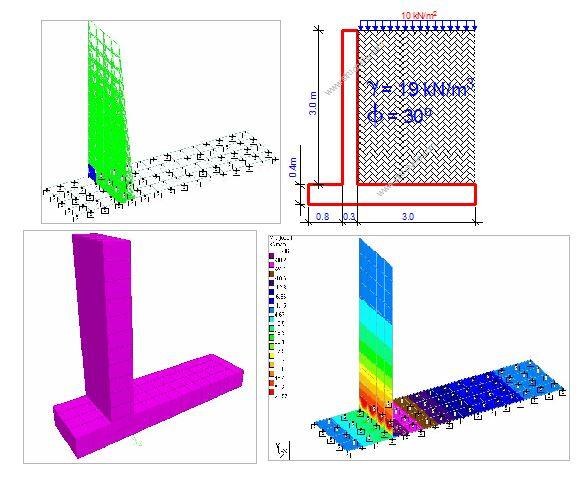
In this post, we are going to show how cantilever retaining walls can be analysed and designed on Staad Pro software, and also compare the answer obtained with classical solutions. We should know that retaining walls must satisfy geotechnical, equilibrium, structural, upheaval, seismic considerations, etc. As a result, the designer must ensure that by appropriate knowledge of materials, site conditions, etc, he/she will provide suitable dimensions of the retaining wall that will ensure resistance of the structure to overturning, sliding, bearing capacity failure, uplift, etc. After appropriate sizing of the retaining wall, the structural analysis and design will commence to determine the action effects (bending moments, shear forces, axial forces, deflection etc), and provision of proper reinforcements to resist the action effects.

In the past, Structville has published a 17 page document on geotechnical design of cantilever retaining walls subjected to earth load, pavement surcharge load, traffic load, etc. This loading situation can be found when retaining wall is used to support embankment carrying traffic road way.  It was interesting to see how Design Approach 1 (DA1) of Eurocode 7 was used to ensure the geotechnical stability of the wall. Just in case you missed it, kindly download the PDF from the link below;

Geotechnical Design of Cantilever Retaining Walls to Eurocode 7

In this post, let us consider the retaining wall sized and loaded as shown in Figure 2. This structure has been modelled on Staad Pro in order to determine the action effects due to the applied load.

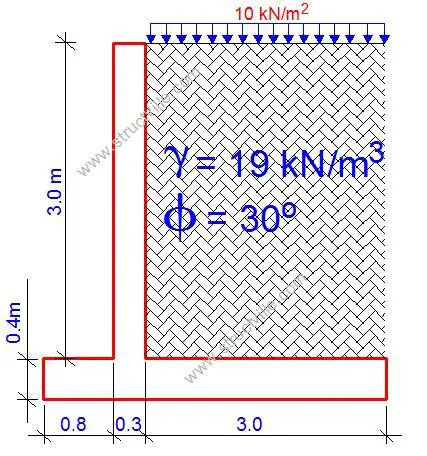Fig 2: Cantilever retaining wall

The retaining wall is subjected to a 3m thick earthfill, and a variable surcharge pressure of 10 kPa. Given that the retained earth has an angle of internal friction of 30°, we can obtain the Rankine active earth pressure as follows;

k _a = (1 – sin 30)/(1 + sin 30) = 0.333

[ Get this publication on Design of Swimming Pools and Underground Water Tanks into your mailbox by clicking HERE or by clicking on the book cover ]

Therefore the actions on the retaining wall that will be input into Staad Pro as are as follows;

Vertical actions
(1) Self weight (to be calculated automatically by Staad)
(2) Weight of earthfill (19 kN/m³ × 3m) = 57 kN/m²
(3) Surcharge load = 10 kN/m²

Horizontal Actions
(4) Triangular earth pressure = (0.333 × 19 × 3) = 18.98 kN/m²
(5) Uniform surcharge pressure = (0.333 × 10) = 3.333 kN/m²

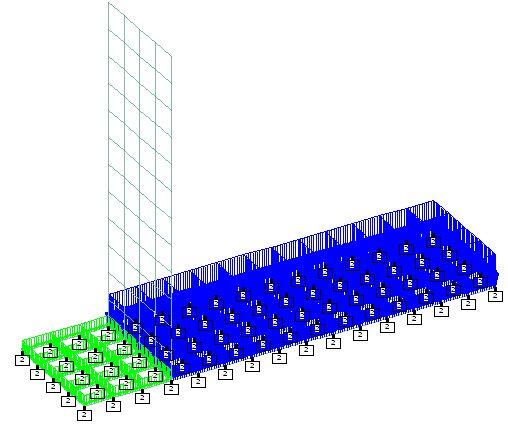
The wall has been modelled per metre run on Staad, and plate mat foundation was utilised with coefficient of subgrade modulus of 100000 kN/m²/m.

Steps to adopt
(1) Model the retaining wall utilising plate element meshing, assign thickness of 0.4m to the base, and 0.3m to the wall. Also assign plate mat foundation of subgrade modulus 100000 kN/m²/m to the base in the y-direction.

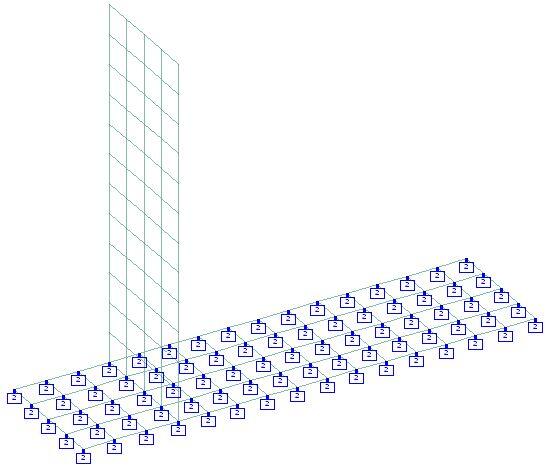Fig 3: Modelling and meshing of the retaining wall

(2) Assign the following loads to the structure

Load Case 1 (LC1)
(a) Self weight to the whole structure
(b) Weight of earth fill to the heel of the retaining wall (57 kN/m²)
(c) Assume that the base is buried 1m into the ground, hence apply vertical pressure load of (19 kN/m²) to the toe but neglect all passive pressures.

Fig 4: Permanent vertical actions on the retaining wall base

[ Get this publication on Design of Residential Buildings Using Staad, Orion, and Manual Calculations into your mailbox by clicking HERE or by clicking on the book cover ]

Load Case 2 (LC2)
(d) Triangular earth pressure to the wall (18.98 kN/m²)

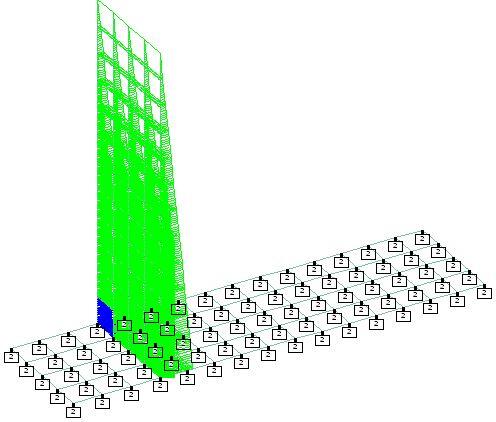Fig 5: Horizontal active earth pressure on the wall

Load Case 3 (LC3)
(e) Uniform horizontal surcharge pressure to the walls (3.333 kN/m²)
(f) Uniform vertical surcharge pressure to the heel (10 kN/m²)

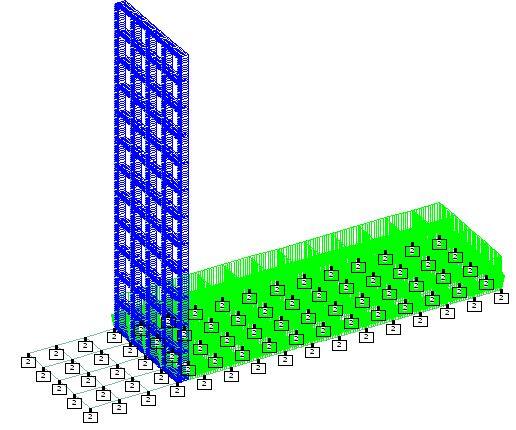Fig 6: Surcharge loads on the wall and on the base

Combination (Ultimate limit state)
p _Ed = 1.35LC1 + 1.35LC2 + 1.5LC3

A little consideration will show that the load cases 1 and 2 are treated as permanent actions, while load case 3 is treated as a variable action.

(3) Analyse the structure for the load cases
(N/B): You may need to increase the iteration limits for the load cases containing horizontal actions to converge

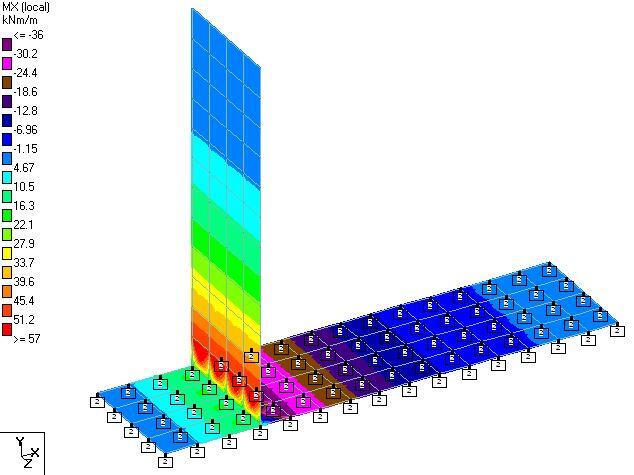Fig 7: Main bending moment on the retaining wall

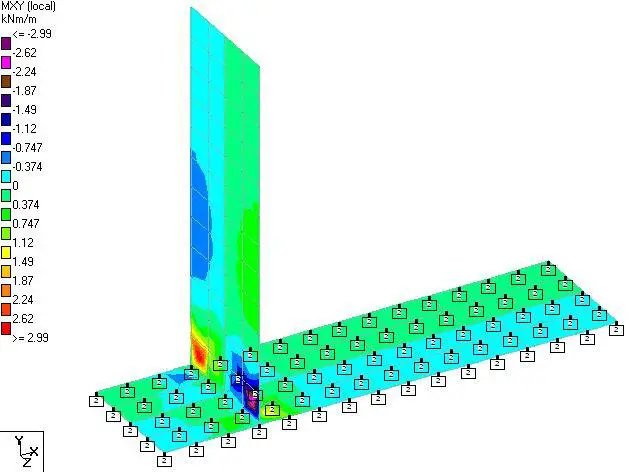Fig 8: Twisting moment on the retaining wall

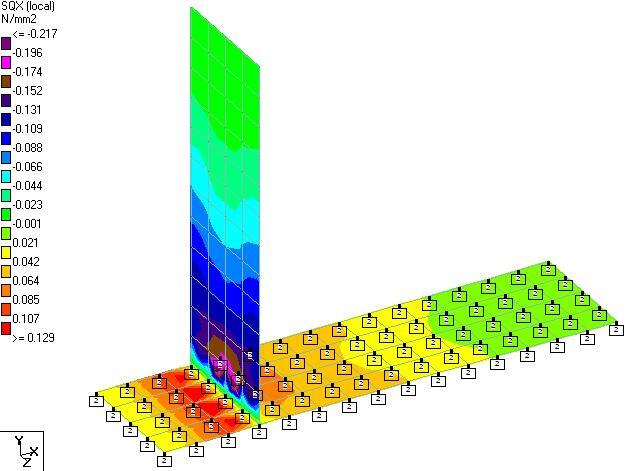Fig 9: Shear stress on the retaining wall

On considering the bending moment diagram Mx (Figure 7), we can see that the maximum moment close to the base of the wall is 57 kNm/m. If we add the effect of torsion (Figure 8), the design moment can be taken as 57 + 2.99 = 59.99 kNm/m.

To carry out manual analysis, we will have to follow the steps given below to obtain the maximum moment at the base of the wall. The actions causing bending on the wall are the horizontal earth pressure and the horizontal surcharge pressure.

Moment from surcharge pressure = [(3.333 × 3²)/2] = 14.998 kNm/m
Moment from horizontal earth pressure = [(18.98 × 3)/2] × (3/3) = 28.47 kNm/m

At ultimate limit state, M_Ed = 1.35(28.47) + 1.5(14.998) = 60.931 kNm/m

A little consideration will show that Staad Pro and Manual calculations gave almost the same value for wall bending moment. However, I expect the value of base moment from Staad Pro to be lower than the one from manual analysis. Kindly verify this at your private time.

Thank you for visiting Structville today and God bless you.

Design of Retaining Wall Using Staad Pro

Source: https://structville.com/2019/08/analysis-and-design-of-cantilever-retaining-walls-on-staad-pro.html

Share this post